This experiment on spherometer is for engineering physics and class 11 physics practical. It is included with spherometer least count, formula for radius of curvature of spherometer, observation table for determination of radius of curvature and important viva questions on this practical.
To determine the radius of curvature of a convex/concave mirror/surface using a spherometer.
Theory
Spherometer is used to measure the thickness or the radius of curvature of a curved surface. The radius of a spherometer is
R=\frac{l^{2}}{6h}+\frac{h}{2}
Where l is the distance between any two legs of the spherometer and h is the perpendicular distance between the legs and screw when it is above the spherical surface
Apparatus
- A spherometer
- A convex/concave mirror/surface
- A plane glass plate
- A plane paper
- A ruler
Procedure
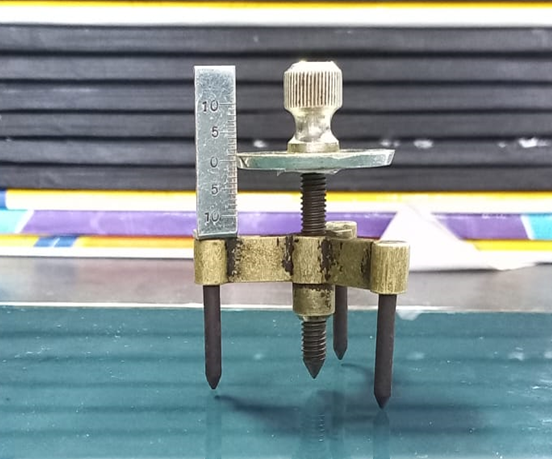
- Raise the screw of the spherometer and press on to the practical notebook to get the marks of the three legs of the spherometer.
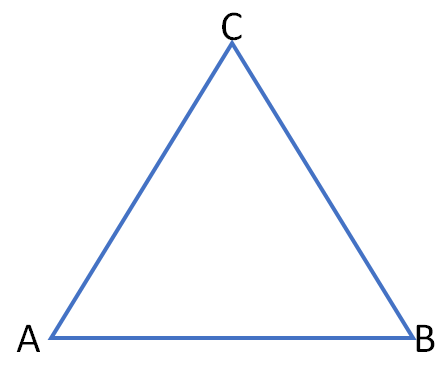
- Name the marks as A, B and C. Join AB, BC and CA and measure the distances. Take the mean of these distances to calculate the value of ‘l’.
- Now measure the screw pitch. Observe the linear scale moves for one complete rotation of the circular scale. Calculate least count by dividing screw pitch to total number of circular scale divisions.
- Now place the convex surface onto the glass plate. Then place the spherometer on the surface of the convex surface or mirror.
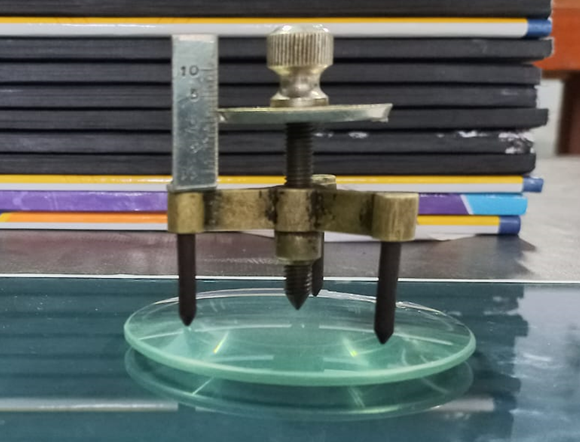
5. Read the circular scale reading when the screw and all three legs touch the convex surface. Make sure that all legs and screw touch the surface by passing a plane paper to the screw head and three legs.
6. Now remove the convex surface and place the spherometer above the plane glass sheet.
7. Count the total number of complete rotations when the spherometer screw touch the glass surface. Also take the reading of circular scale.
Results
Distance between the two legs of the spherometer
A, B and C are legs of the spherometer. The average distance between the two legs are
AB = _____ cm
BC = _____ cm
CA= _____ cm
Mean value of length l=\frac{AB+BC+CA}{3} cm=_____ cm
Least Count of spherometer
Screw pitch (p) = _____ mm
Total number of circular scale divisions, N = _____
Least count, (L.C.) = \frac{Screw \hspace{0.2cm}pitch}{Total \hspace{0.2cm} number \hspace{0.2cm} of \hspace{0.2cm} circular \hspace{0.2cm} scale \hspace{0.2cm} divisions}
Least count, (L.C.) =____ mm
Table for determination of h
| No. of Obs. | Circular Scale reading on convex surface (a) | Circular Scale reading on plane glass surface (b) | No. of complete rotations (n) | No. of circular scale divisions in incomplete rotations (x = a \sim b) | Total Reading = (n \times p)+ (x \times L.C) mm | Mean h mm |
| 1 | ||||||
| 2 | ||||||
| 3 | ||||||
| 4 | ||||||
| 5 |
Calculations
R=\frac{l^{2}}{6h}+\frac{h}{2}
R = ____ mm = ______ cm
So, the radius of curvature of the given convex surface is _____ cm
Precautions and Discussions
- The screw should be moved in the same direction to avoid back-lash error of the screw.
- Excess rotation should be checked and avoided.
- The screw should rotate without any friction.
Viva questions on Spherometer experiment for class 11 and engineering physics practical
- What is the main principle behind the working of a spherometer?
Answer: The spherometer works on the principle of micrometer screw. - Why this instrument is called spherometer?
Answer: It is called spherometer because it measures the radius of curvature of a spherical surface. - Write down the formula for determination of radius of curvature of a spherical surface using spherometer?
Answer: The radius of curvature of a spherical surface is given by
R=\frac{l^{2}}{6h}+\frac{h}{2}
Where l is the distance between any two legs of the spherometer and h is the perpendicular distance between the legs and screw when it is above the spherical surface - What is the value of radius of curvature of a plane surface?
Answer: The radius of curvature of a plane surface is infinite. - What is the pitch of a spherometer?
Answer: The pitch is defined as the linear distance travelled by the circular disc in one complete rotation. - Write down the lens makers formula.
Answer: The lens makers formula is given by
\frac{1}{f}=[\frac{\mu_{2}}{\mu_{1}}-1][\frac{1}{R_{1}}-\frac{1}{R_{2}}] - Can you measure the focal length of a lens by using spherometer.
Answer: Yes, by measuring the radius of curvatures and putting these values in lens makers formula. - Why good spherometers are made of gun metal?
Answer: To minimize the wear and tear.
Note: Gun metal is an alloy of Copper, tin and Zinc.